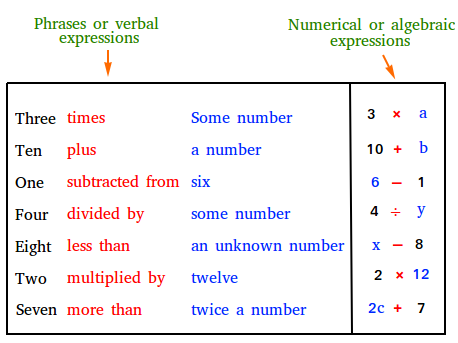
Algebra 1 requires of students to learn on how to compos expressions. As such, this comprehensive blog delves on a journey to understand the art of how-to compos expressions, exploring the building blocks, rules, and practical applications. So, by the end of this exploration, you’ll be equipped with the skills to construct and decipher algebraic expressions with confidence.
Therefore, Learnerscamp has come a long way to help students understand different mathematical concepts including How to Compos Expressions. Not excluding other maths topics such as area, perimeter, coefficients and How to calculate; Expressions, Variables, and Operations in Algebra among many other topics. Learnerscamp also enables you to learn maths at the comfort of your space, pace and time.
Understanding how to compos expressions:
Composing expressions involves combining numbers, variables, and operations to represent mathematical relationships or real-world scenarios. Hence, it’s like piecing together a puzzle where each element contributes to the overall picture. Therefore, let’s dive deeper into the process of composing expressions with practical examples:
Example 1: Consider the expression representing the total cost of purchasing x items, each priced at $y.
- Expression: Total Cost = x * y
- Here, “x” represents the number of items, and “y” represents the price per item.
- If you’re buying 5 items priced at $10 each, the expression becomes: Total Cost = 5 * 10 = $50.
Example 2: Explore the expression representing the area of a rectangle with length “L” and width “W.”
- Expression: Area = L * W
- Here, “L” represents the length, and “W” represents the width.
- If the length is 8 units and the width is 6 units, the expression becomes: Area = 8 * 6 = 48 square units.
Components of Composing Expressions:
- Numbers: Numerical values that represent quantities or measurements. Notably, these can be constants or coefficients in expressions.
- Variables: Symbols that represent unknown or changing quantities. Thus, variables allow us to generalize mathematical relationships and solve problems efficiently.
- Operations: Mathematical operations such as addition, subtraction, multiplication, division, and exponentiation. As such, these operations dictate how the components of expressions are combined.
Rules for Composing Expressions:
- Follow the order of operations (PEMDAS/BODMAS): Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right).
- Apply the distributive property when necessary to simplify expressions.
- Combine like terms by adding or subtracting coefficients of similar variables.
Practical Applications of Composing Expressions:
- Finance: Compose expressions to calculate total expenses, profits, or savings based on various factors such as prices, quantities, and discounts.
- Geometry: Compose expressions to find the perimeter, area, or volume of geometric shapes such as rectangles, triangles, or cylinders.
- Physics: Compose expressions to represent physical laws or formulas, such as Newton’s second law (F = m * a) or the equation for kinetic energy (KE = 0.5 * m * v^2).
Example 3: Consider the expression representing the volume of a rectangular prism with length “l”, width “w”, and height “h.”
- Expression: Volume = l * w * h
- If the length is 4 units, width is 3 units, and height is 5 units, the expression becomes: Volume = 4 * 3 * 5 = 60 cubic units.
Example 4: Explore the expression representing the total distance traveled by a car moving at a constant speed “s” for time “t” hours.
- Expression: Total Distance = s * t
- If the car travels at a speed of 60 miles per hour for 2 hours, the expression becomes: Total Distance = 60 * 2 = 120 miles.
Conclusion:
Conclusively, composing expressions is an essential skill in Algebra 1, allowing us to model mathematical relationships and solve real-world problems. Thus, by understanding the components, rules, and practical applications of composing expressions, you’ve embarked on a journey of algebraic exploration. Embrace the challenges, practice diligently, and let the art of composing expressions empower you to navigate the complexities of algebra with confidence.