Understanding the relationship between speed, time, and distance is fundamental in many aspects of life, from everyday tasks to advanced scientific explanations. Whether you’re planning a road trip, analyzing motion in physics, or even managing logistics in business, the ability to calculate these parameters accurately is crucial. In this blog post, Learnerscamp will help you delve into the basics of how to calculate speed, time, and distance, providing you with the tools to tackle a wide range of problems with confidence.
Therefore, Learnerscamp has come a long way to help students understand different mathematical concepts including How to Compos Expressions. Not excluding other maths topics such as area, perimeter, coefficients and How to calculate; Expressions, Variables, and Operations in Algebra among many other topics. Learnerscamp also enables you to learn maths at the comfort of your space, pace and time.
Understanding the Concepts
- Speed: Speed is the rate at which an object covers distance. It is typically measured in units such as meters per second (m/s) or kilometers per hour (km/h).
- Time: Time represents the duration of an event or the interval between two points in time. It is measured in units such as seconds, minutes, hours, etc.
- Distance: Distance is the amount of space between two points. It can be measured in units such as meters (m), kilometers (km), miles (mi), etc.
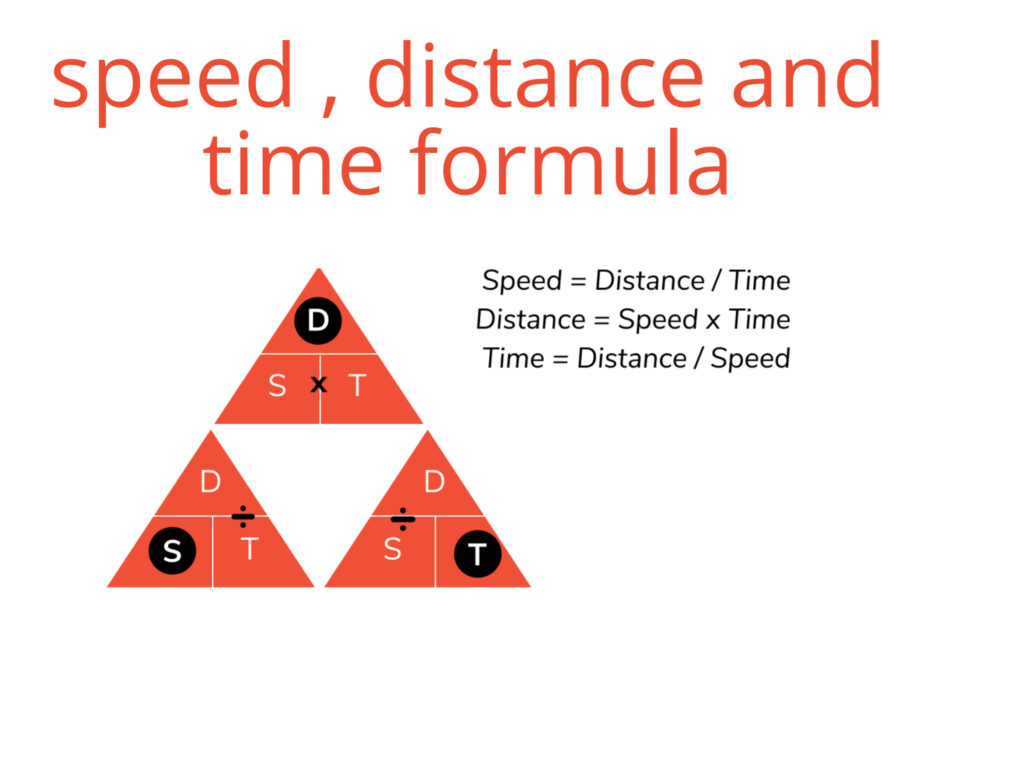
calculating speed, time, and distance
- Speed = Distance / Time This formula calculates the speed of an object given the distance it travels and the time it takes to travel that distance.
- Time = Distance / Speed This formula calculates the time taken to cover a certain distance at a given speed.
- Distance = Speed × Time This formula calculates the distance covered by an object traveling at a certain speed for a specific duration.
Example Problems:
Problem 1: If a car travels a distance of 300 kilometers at an average speed of 60 km/h, how long does it take to reach its destination?
Solution: Using the formula Time = Distance / Speed, Time = 300 km / 60 km/h Time = 5 hours
Therefore, it takes the car 5 hours to reach its destination.
Problem 2: An athlete runs at a speed of 8 meters per second. How far can the athlete run in 25 seconds?
Solution: Using the formula Distance = Speed × Time, Distance = 8 m/s × 25 s Distance = 200 meters
Therefore, the athlete can run a distance of 200 meters in 25 seconds.
Conclusion: Mastering the calculations of speed, time, and distance opens up a world of possibilities in various fields of study and everyday life. By understanding the basic concepts and applying the appropriate formulas, you can solve a wide range of problems efficiently and accurately. So, whether you’re planning a journey, analyzing motion, or simply curious about the world around you, remember that the principles of speed, time, and distance are always at your disposal.








