Fractions are essential mathematical tools used to represent parts of a whole or quantities expressed as ratios. From basic arithmetic to complex problem-solving, understanding how to manipulate fractions is crucial for navigating various mathematical scenarios. Thus, this blog explores the intricacies of fraction calculations, focusing on addition, subtraction, multiplication, division, and more. Hence, by the end of this exploration, you’ll be equipped with the knowledge and skills to confidently perform fraction calculations and tackle a wide range of mathematical challenges.
Before we go deeper into exploring integers and number lines, I would like to remind you as a learner that LearnersCamp provides additional insights into these disparities. Additionally, LearnersCamp has grown to become a valuable platform for learners seeking to deepen their understanding of mathematical concepts. Moreover, it has a user-friendly interface and a vast array of educational materials, including articles, tutorials, and interactive quizzes; LearnersCamp offers comprehensive guidance on such topics. Hence, by visiting LearnersCamp, learners can access clear explanations, practical examples, and engaging exercises to solidify their grasp of these fundamental mathematical principles.
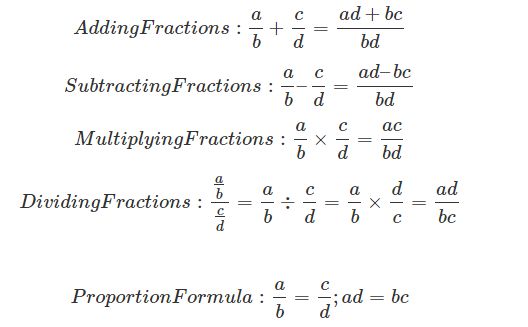
Understanding Fraction Addition and Subtraction:
Fraction addition and subtraction involve combining or separating parts of a whole. Let’s delve into these operations:
- Addition of Fractions:
When adding fractions, ensure they have the same denominator. Add the numerators and keep the denominator unchanged. Example:
3/4 + 2/4 = 5/4 (Since the denominators are the same, simply add the numerators.) - Subtraction of Fractions:
Similar to addition, subtract fractions with the same denominator by subtracting the numerators while keeping the denominator unchanged. Example:
5/6 – 2/6 = 3/6 (Again, since the denominators match, subtract the numerators.)
Reviewing Least Common Multiple (LCM) and Equivalent Fractions:
The LCM is crucial for finding a common denominator when adding or subtracting fractions. It’s the smallest common multiple of two or more numbers. Equivalent fractions have the same value but different numerators and denominators.
Practical Illustration:
Consider adding 1/3 and 1/6. The LCM of 3 and 6 is 6. Convert both fractions to have a denominator of 6: 2/6 + 1/6 = 3/6, which simplifies to 1/2.
- Multiplication of Fractions:
To multiply fractions, multiply the numerators together and the denominators together.
Example:
2/3 x 3/5 = (2 x 3) /(3 x 5) = 6/15
- Practical Illustration:
Imagine baking cookies. If a recipe calls for 2/3 cup of flour and 3/5 cup of sugar, you’d need 6/15 cups of flour and sugar combined.
- Division Involving Fractions:
To divide fractions, multiply the first fraction by the reciprocal of the second fraction.
Example:
(⅔) / (4/5) = {2/3} x (5/4) = 10/12
- Practical Illustration:
Imagine sharing 2/3 of a pizza equally among 4/5 of your friends. Each friend gets 10/12 of the pizza.
Division Involving Mixed Numbers:
When dividing mixed numbers, convert them to improper fractions, then follow the same procedure as dividing fractions.
Example:
3 (½) / 1 (¼) can be converted to (7/2) / (5/4).
Sums and Differences Involving Positive and Negative Fractions:
Adding or subtracting positive and negative fractions follows the same rules as integers. Different signs indicate subtraction, while the same signs indicate addition.
- Practical Illustration:
Consider adding 1/4 and -2/5. Since they have different signs, subtract their absolute values: 1/4 – 2/5 = 5/20 – 8/20 = -3/20.
Mastering fraction calculations is essential for tackling a variety of mathematical problems and real-life scenarios. thus, by understanding addition, subtraction, multiplication, and division of fractions, as well as working with mixed numbers and positive and negative fractions, you’ll be equipped to confidently navigate numerical challenges. additionally, we’ve explored the intricacies of fraction calculations, reinforcing key concepts and techniques through practical illustrations and examples, .So, whether you’re dividing ingredients for a recipe, solving business problems, or analyzing data, remember to leverage the power of fractions to achieve accurate and meaningful results